An engineering company is about to undertake a major overhaul of a factory’s machinery for a customer. The overhaul will be carried out on a Saturday and Sunday, but, if it is not completed by the Monday morning, the factory will experience serious production losses. In this event, the engineering company has agreed to compensate the customer by paying a penalty of $20 000. The manager of the engineering company has to decide how many engineers to include in the overhaul team. Each engineer in the team will be paid $480 for working over the weekend, but because of the nature of the work, only teams of 10, 15 or 20 engineers can be considered. The manager estimates that the chances of a 10-person team completing the overhaul by the Monday morning is only 0.4. A 15-person team has, he estimates, a 0.6 probability of meeting the deadline, while a 20-person team has a 0.9 probability of completing the work in time for Monday morning.
(a) Assuming that the manager wants to minimize expected costs, how large a team should he choose?
(b) Having made a provisional decision about the size of the team, the manager hears that a piece of specialized equipment will be available for hire on the Sunday, at short notice. The cost of hiring this equipment would be $4400, and it would require at least 15 engineers to operate it. However, it is virtually certain that the overhaul would be completed on time if the equipment was used. Before making a decision on whether to hire the equipment, the manager will review the progress that has been made on the Saturday evening. He reckons that there is a 0.5 probability that a 15-person team would be behind schedule by Saturday evening, while there is only a 0.2 probability that a 20-person team will be in this position. He then calculates the probabilities of the overhaul overrunning the deadline if the equipment is not hired, given the position on Saturday evening. These probabilities are shown below:
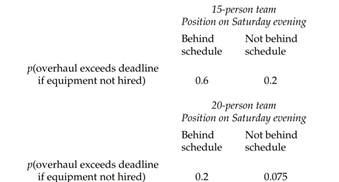
How many people should the manager now include in the team and should he hire the equipment on the Saturday evening?

