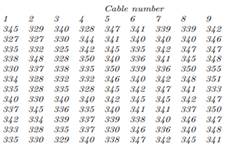
A high voltage electric cable consists of 12 wires. The following data are the tensile strengths of the 12 wires in each of 9 cables. (a) Use a computer to draw side-by-side box plots for the nine cables. Do the box plots indicate that the strength of the wires varies among the cables? (b) Cables 1 to 4 were made from one lot of raw material and cables 5 to 9 came from another lot. Do the box plots indicate that the tensile strength depends on the lot? (c) Perform a one–way ANOVA to test the null hypothesis that the tensile strength does not vary among the cables. (d) Use Tukey’s method to determine those differences in the treatment means that are significant. Are these results consistent with the information that cables 1 to 4 came from one lot of raw material and cables 5 to 9 came from another lot? (e) Express the comparison between the two lots as a contrast, and test whether the tensile strength depends on the lot.

(Source: A. Hald (1952), Statistical Theory with Engineering Applications, John Wiley & Sons, New York. Used with permission.)