Determine the area of the ellipse of problem 7-4.

Problem 7.4

An ellipse is a plane figure described by the locus of a point P(x, y) that moves such that the sum of its distances from two fixed points (foci) is a constant. If the ellipse has foci located at A (-c, 0) and B (c, 0) and the distance ACB is 2a, then by setting b = Jn, the equation of the ellipse is simplified to

(a and b are termed the semiaxes of the ellipse).

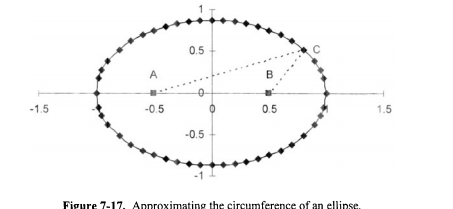
For the ellipse shown in Figure 7-17, with foci at x = -0.5, y = 0 and x = 0.5, y = 0 and a = 1, determine the circumference of the ellipse.