Q1:
In a deep ocean with constant salinity 3.5% the water temperature distribution
is assumed to be exponential in depth,
![]()
where T and T0 are in degrees Celsius, and z is in meters.
a. What is the minimum surface water temperature for which a deep sound channel
(SOFAR) will exist?
b. Determine the depth of the channel axis and the associated sound speed as function
of the surface temperature.
Q2:
Consider a 4000-m deep ocean with constant salinity 3.5%. The water temperature
distribution is assumed to be exponential in depth,
![]()
where T is in degrees Celsius, and z is in meters.
a. At approximately which latitude would you expect to find such an environment?
b. For a source at 100m depth, discuss the existence of the various ray paths (RR,
RSR, RBR and SRBR) in this environment.
c. What is the surface temperature for which no pure RSR and RBR paths exist?
Q3:
In air acoustics, the conventional reference for decibels is dB re 0:0002 dyn/cm2 as opposed to dB re 1_Pa used in ocean acoustics.
a. A human whisper and shout have acoustics powers of about 10_10 and 10_5 watts, respectively. Express their dB levels using both conventions. What would be the dB levels if the whole world shouted at once (in the same place)? Compare that to a jet or rocket in air or various types of ships in water.
b. If a rock band played at the pain threshold, of about 140 dB, what is its power
output in watts. What is its corresponding sound pressure level in water?
c. For a 120-dB source in water (measured one meter from the source), what would its dB level be at ranges 1, 10, and 100km assuming spherical spreading; cylindrical spreading? The loudest whales have source levels of about 190 dB. Compare this to a rock band.
Q4:
An omni-directional source of frequency f is placed at a distance zs from an infinitely rigid wall bounding a fluid halfspace with constant sound speed c.
a. Describe the radiation pattern in the limit of ![]()
b. Derive the expression for the number of Lloyd-mirror beams.
c. Derive the asymptotic field decay parallel to the wall, and compare to the corresponding
pressure-release surface result.
Q5:
Estimate the convergence zone (CZ) separation for an Arctic environment with the sound speed profile given below. Assume linear sound speed variation between the profile depths.
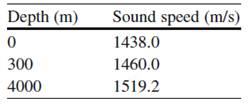
Q6:
Write a program for computing and displaying the magnitude and phase of the reflection and transmission coefficients for the interface separating two fluid halfspaces.
a. Use your code to illustrate the concept of a critical angle by properly choosing the sound speeds and densities.
b. For grazing angles of incidence smaller and larger than critical, discuss the depth-dependence (direction perpendicular to the interface) of the reflected and transmitted fields.
c. Discuss the behavior of the phase of the reflection coefficient for incident grazing angles less than and larger than critical.
d. Create an example illustrating the concept of an intromission angle.
Q7:
Derive the expression for the reflection coefficient for a fluid layer overlying an infinitely rigid halfspace. Give a physical explanation for the frequency and grazing angle dependence of the magnitude and phase.

