Refer to the part of Example 7.20 (pp. 236–237) in which two students are drawn without replacement from a class of 30 students in which three are left-handed. Draw a tree diagram to illustrate that the probability of selecting two lefthanded students is 1/145.

Example 7.20

Choosing Left-Handed Students A class of 30 children includes 27 who are righthanded and 3 who are left-handed. The teacher plans to randomly choose one child on Monday and one on Tuesday to help demonstrate a science experiment. The teacher, who is left-handed herself, enjoys it when a left-handed assistant is chosen. She wonders whether there is a higher probability of choosing left-handed students on both Monday and Tuesday if she samples with replacement or without replacement. Here is how you would find the probability that a left-handed student is drawn on both days, when sampling with and without replacement:

Sampled with replacement:

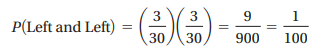
Sampled without replacement:

When sampling without replacement the probability that the second student is lefthanded, given that the first student was left-handed, is a conditional probability. It is found by noticing that there are 29 students remaining, of whom 2 are left-handed. Note that there is a higher probability of choosing a left-handed student both days if sampling is with replacement. This makes sense because the conditional probability of choosing a left-handed student Tuesday is higher when the left-handed student chosen Monday remains in the pool of possible choices.