Brooks Development Corporation (BDC) faces the following capital budgeting decision. Six real estate projects are available for investment. The net present value and expenditures required for each project (in millions of dollars) are as follows:

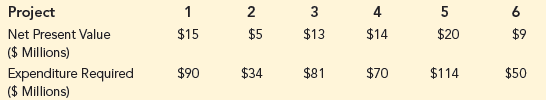
There are conditions that limit the investment alternatives:

••At least two of projects 1, 3, 5, and 6 must be undertaken.

••If either project 3 or 5 is undertaken, they must both be undertaken.

••Project 4 cannot be undertaken unless both projects 1 and 3 also are undertaken.

The budget for this investment period is $220 million.

a. Formulate a binary integer program that will enable BDC to find the projects to invest in to maximize net present value, while satisfying all project restrictions and not exceeding the budget.

b. Solve the model formulated in part a. What is the optimal net present value? Which projects will be undertaken? How much of the budget is unused?