1. Solve the equation 3^x = 81.
a. {27}
b. {5}
c. {4}
d. {3}
2. Write log ₂ (⁷√m ³√n)/k² as the sum and/or difference of logarithms. Express powers as factors.
a. (1/7) log ₂ m + (1/3) log ₂ n – 2log ₂ k
b. (1/7) log ₂ m ⋅ (1/3) log ₂ n ÷ 2log ₂ k
c. 7log ₂ m + 3log ₂ n – 2log ₂ k
d. (7/2) log ₂ m + (3/2) log ₂ n – (2/2) log ₂ k
3. The function f(x) = 6x – 1 is one-to-one. Find its inverse.
a. f ^-1(x) = (x/6) – 1
b. f ^-1(x) = (x – 1)/6
c. f ^-1(x) = (x + 1)/6
d. f ^-1(x) = (x/6) + 1
4. Change the exponential expression 5^-3 = 1/125 to an equivalent expression involving a logarithm.
a. log _-3 1/125 = 5
b. log ₅ 1/125 = -3
c. log ₅ -3 = 1/125
d. log _1/125 5 = -3
5. Write log ₇ √(xy)/15 as the sum and/or difference of logarithms. Express powers as factors.
a. (1/2) log ₇ xy – (1/2) log ₇ 15
b. (1/2) log ₇ x ⋅ (1/2) log ₇ y ÷ (1/2) log ₇ 15
c. (1/2) log ₇ x + (1/2) log ₇ y – (1/2) log ₇ 15
d. (1/2) log ₇ x + (1/2) log ₇ y – log ₇ 15
6. The value of a particular investment follows a pattern of exponential growth. You invested money in a money market account. The value of your investment t years after your initial investment is given by the exponential growth model A = 5100e^0.047t. How much did you initially invest in the account?
a. $2550.00
b. $5345.42
c. $5100.00
d. $239.70
7. Solve the equation log ₄ (x + 4) + log ₄ (x – 2) = 2
a. {-6}
b. {4}
c. {4, -6}
d. {5}
8. Find the exact value of the logarithmic expression log ₄ 1/64.
a. -1/3
b. -3
c. 1/3
d.
9. Find the amount that results from the investment of $480 invested at 6% compounded quarterly after a period of 3 years.
a. $573.90
b. $93.9
c. $565.42
d. $571.69
10. Solve the equation log ₄ (x + 2) = -1
a. {-7}
b. {-7/4}
c. {9/4}
d. {9}
11. The graph of a logarithmic function is shown. Select the function which matches the graph.

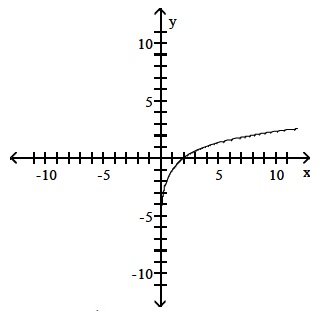
a. y = log ₂ (x + 1)
b. y = log ₂ (x – 1)
c. y = log ₂ x
d. y = log ₂ x – 1
12. Find the inverse of the function and state its domain and range.
{(-3, 4), (-1, 5), (0, 2), (2, 6), (5, 7)}
a. {(3, 4), (1, 5), (0, 2), (-2, 6), (-5, 7)}; D = {3, 1, 0, -2, -5}; R = {2, 4, 5, 6, 7}
b. {(-3, -4), (-1, -5), (0, -2), (2, -6), (5, -7)}; D = {-3, -1, 0, 2, 5}; R = {-7, -6, -5, -4, -2}
c. {(4, -3), (5, -1), (2, 0), (6, 2), (7, 5)} D = {2, 4, 5, 6, 7}; R = {-3, -1, 0, 2, 5}
d. {(3, -4), (1, -5), (0, -2), (-2, -6), (-5, -7)}; D = {3, 1, 0, -2, -5}; R = {-7, -6, -5, -4, -2}
13. Find the domain of the composite function f ∘ g if f(x) = 28/x and g(x) = -8/(x – 7).
a. {x | x ≠ 7}
b. {x | x ≠ 0, x ≠ 7, x ≠ 4}
c. {x | x ≠ 7, x ≠ 0}
d. {x | x is any real number}
14. Express 6log _b m – log _b n as a single logarithm.
a. log _b m⁶ ÷ log _b n
b. log _b (m⁶ – n)
c. log _b (m⁶/n)
d. log _b (6m/n)
15. In 1990, the population of a country was estimated at 4 million. For any subsequent year the population, P(t) (in millions), can be modeled by the equation P(t) = 240/(5 + 54.99e^-0.0208t), where t is the number of years since 1990. Estimate the year when the population will be 21 million.
a. approximately the year 2093
b. approximately the year 2041
c. approximately the year 2088
d. approximately the year 2016
16. If 5^x = 6,what does 5^-2x equal?
a. 1/36
b. 36
c. 1/12
d. -36

17. For the functions f(x) = x² + 4 and g(x) = x² + 1, find the composite function (f ∘ g)(x).
a. x⁴ + 5
b. x⁴ + 17
c. x⁴ + 2x² + 5
d. x⁴ + 8x² + 17
18. A size 6 dress in Country C is size 52 in Country D. A function that converts dress sizes in Country C to those in Country D is f(x) = 2(x + 20). Find a formula for the inverse of the function described.
a. f ^-1(x) = (x – 20)/2
b. f ^-1(x) = x – 20
c. f ^-1(x) = (x/2) – 20
d. f ^-1(x) = (x/2) + 20
19. If f(x) = 4^x and g(x) = 12, find the point of intersection of the graphs of f and g by solving f(x) = g(x). Give an exact answer.
a. (log ₄ 12, 12)
b. (log ₄ 12, 4)
c. (log ₄ 12, 0)
d. (12, 12)
20. Find functions f and g so that f ∘ g = H(x) = (5 – 2x³)².
a. f(x) = 5 – 2x³ ; g(x) = x²
b. f(x) = (5 – 2x)³ ; g(x) = x²
c. f(x) = x² ; g(x) = 5 – 2x³
d. f(x) = x³ ; g(x) = (5 – 2x)²