The following equations from Example 12.4 give the components of M after an α pulse (assuming the system is in equilibrium just before the α pulse):

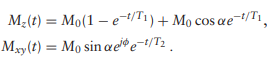
Suppose that we now excite the sample with a train of α pulses, separated by a time T_R. The equilibrium condition is true when T_R is long compared with T₁ and we can assume that M_Z just before the pulse is equal to

Mo. Derive a more general formula for M_Z (t). You can assume that the transverse magnetization has completely dephased before each RF pulse, that is, M_xy(T_R) = 0. (Hint: In this more general formula, M_O will be replaced with the steady-state value of the longitudinal magnetization. Define M_Z after the (n + 1)^th pulse to be  , and M_Z after the n^th pulse to be  . Relate these two quantities with an equation. Derive another (very simple) equation from the steady-state condition. You now have enough information to solve the problem.)