A light plane uniform square lamina of side 2a is mounted in a vertical plane and is free to rotate about an axis through its centre and perpendicular to its plane. Initially two of the edges of the lamina are horizontal. A particle of mass km, k > 0, is attached to the centre of the upper edge and particles of mass m are attached to each of the two bottom corners.

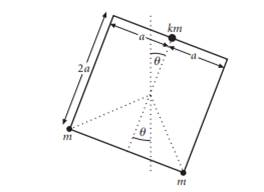
i) By considering a rotation through an angle  of the lamina show that the potential energy of the system relative to the centre of the lamina is mga(k – 2) cos 
. ii) Locate any positions of equilibrium.
iii) Discuss the dependence of the nature of the stability of any equilibrium positions on the value of k.
iv) Given that k = 1 and the mass of the lamina can be neglected write down the energy equation. By differentiating this equation with respect to time find the period of small oscillations of the lamina about the position of stable equilibrium.